Prisms: Definition, Properties, Types, Surface Area, Volume, Examples

Prisms has many types depends on the base. There are many daily things in prisms such as aquarium, tent, tissue box, cupboard, etc..

Definition of Prisms

Prisms is three-dimensional shape that is bordered by two bases and lateral planes.

Number of lateral planes is same with number of edges of the base. Consequently, type of prisms depends on the base.

Properties of Prisms

There are some properties of prisms:

• It has two bases that are congruent and parallel.
• The bases are linked by lateral side/plane.
• Number of lateral sides depends on type of polygon as the base.
• Height of prism is distance between two bases.
• The vertices of prism are twice of vertices of base.
• The edges of prism are three times of the edges of base.

Types of Prisms

There are some types of prism depends on the shape of the base.

• Irregular prism

Irregular prism means prism that has irregular shape as the base. Besides that, irregular prism has irregular cross section in shape.

• Regular prism

Regular prism means prisms that has polygon as the base. In other words, regular prism has regular shape (same measure in length and the angles) in the cross section. It is like triangular prisms (triangle base), square prisms (square base), pentagonal prisms (pentagon base), etc.. It is easier to determine the surface area and volume than the irregular ones.

Surface Area of Prisms

Surface area of prims means total area that is covered the prism. Because of in general concept prism consist of two bases and some faces, then

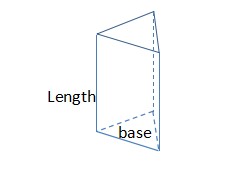
Surface area = 2 x area of base + base perimeter x length

Volume of Prisms

Prisms has some type depends on the base. Volume of prism means capacity in the prism. The volume of prisms in general form is

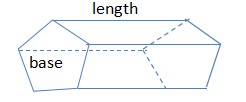
Volume of prism = Area of base x length

Note: base is not always in bottom side. Base is parallel planes.

Right and Oblique Prisms

There are two type of prism depends on the prism’s position (slope).

• Right prism

Right prism is a prism that has aligned edges perfectly.

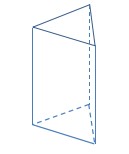
• Oblique prism

Oblique prism is a prism that the edges are not align. The length of oblique prism is distance between base and the center of the top side.

Examples

1. Determine the surface area and the volume if there is a hexagonal prism that has base area 24√3, base perimeter 24 and the height is 8.

2. Determine the volume

Answer

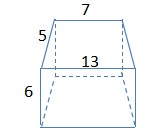
The base is trapezoid, then the height of trapezoid can be calculated using Pythagorean theorem.

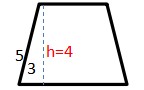
• base area = {(a+b)/2} x height of trapezoid

= {(7+13)/2} x 4

= 10 x 4

= 40

• Volume = base area x height of prism

= 40 x 6

= 240

3. Determine the height of triangular prism if the volume is 84 cm³ and the base is triangle with 3, 4, and 5 cm as the sides.