Square is one of common things in daily life. There are many square things. They are chess board, clock, crackers, origami paper, etc..

Table of Contents

Definition of Square

Square is specific shape of rectangle. It is because square has right angles in all of the corner. But it has same length in all of the sides.

Besides that, square is a parallelogram then it has two pairs of parallel sides. They are opposite sides that parallel each other.

Square is also rhombus. It is because square has four same length sides. But the different is the corner. Rhombus have not right angles in every corner.

Area of a Square

Area of a square means two-dimensional plane that is bordered by four same length sides. It is calculated by multiplying the sides.

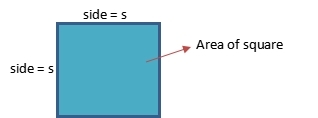
Area = A = side x side = s^²

Perimeter of a Square

Perimeter of a square means sum of the sides. Because of it has four same length sides, it can be written as four times the sides.

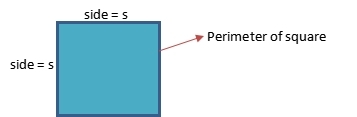
Perimeter = P = side + side + side + side = 4 x sides = 4s

Diagonal of a Square

Diagonal of a square means a line that connect one of the corners with the opposite. Square has two same length diagonals. Beside that the diagonals intersect in middle line then it divides square to be four isosceles right triangles.

Calculating diagonal of the square is same with calculating diagonal of a rectangle. It starting by using Pythagorean theorem but in the last it has specific formula.

diagonal = d = √(s^² + s^²) = √(2s^²) = s√2

By using diagonal concept, it can be formulated to determine the area of square.

Area = A = d^² / 2

Properties of a Square

There are properties of square:

It has four same length sides
It has four right angles (in every corner)
Diagonals of square are congruent.
Diagonal divide the corner (right angle) to be 45°.
Two diagonals divide the square to be four isosceles right triangles.

Examples

Determine the difference of area and perimeter of the square if the side = 6 cm.

Answer

Side = 6 cm

Area = s² = 6² = 36 cm²

Perimeter = 4 x s = 4 x 6 = 24 cm

Then the difference is 36 – 24 = 12 cm.

Remember that difference is always positive.

Another answer of difference is 24 – 36 = -12 because of it is difference then the right answer is 12 cm (positive).

2. There is a yard in square shape. The area is 144m². How far the distance from the corner to the opposite?

Answer

Area of the yard = 144 m²

Distance from the corner to the opposite is diameter.

Then,

Area = 144

Area = s²

144 = s²

s = 12 m

so, the distance from the corner to the opposite (diagonal) is s√2 = 12√2 m.

3. There is a square that has 56 cm as the perimeter. Determine the diagonal of the square.

Answer

Perimeter = 56

First step is determining the side.

P = 56

P = 4 x s

56 = 4 x s

s = 56 / 4

= 14 cm

Then perimeter = s √2 = 14√2 cm.